Physics – Page 2 – Ben Craven

Walking bass

How fast does a plucked guitar string move? It’s a complete blur, so surely it’s travelling at a terrific speed. 50 miles per hour? 100 miles per hour? What do you think?

"Vibrating guitar string" by jar [0] is licensed under CC BY 2.0 — “Vibrating guitar string” by jar[0]. See end of post for full attribution.

Walking pace. A typical speed for the middle of a guitar string given a good twang is walking pace. And that’s the middle of the string. Near its ends, it’ll be moving much more slowly.

How can that be so? Well, although the string is going back and forth hundreds of times a second, it’s only travelling a few millimetres on each trip. So the distance that it travels in each second isn’t as much as you might expect. It certainly isn’t as far as I expected.

If you think that the string moves slowly, what about the body of the guitar? The string itself radiates very little sound into the air; its job is to set the body of the guitar vibrating. The body of the guitar, with its much larger area, is much more effective than the string at setting air into motion. Yet we can’t even see the body vibrating. At what snail’s pace must it be moving?

Remember also that the air molecules on which the guitar body acts are already travelling at something like 500 metres per second. Isn’t it astonishing that the sub-pedestrian movements of the guitar affect the movement of the air molecules enough to produce a sound that we can easily hear?

The calculation

Suppose that we look near the centre of the string, where its movement is the greatest. Shortly after being plucked, the width of the blur that we see is going to be something like 5 mm. So for every complete oscillation, the string does a round trip of about 10 mm.

The frequencies of the strings on a standard 6-string acoustic guitar are (to the nearest whole number) 82, 110, 147, 196, 247 and 330 hertz (one hertz is one oscillation per second). If we multiply these frequencies by the 10 mm round trip, it tells us how far the centre of each string travels in one second, that is, its average speed. I’ve converted these speeds into metres per second. For comparison, a brisk walk at 4 mph is about 1.8 ms^-1.

Note name	Mean speed of middle of string in metres per second (to 2 sig. fig.)
E	0.82
A	1.1
D	1.5
G	2.0
B	2.5
E	3.3

Our brisk walk is right in the middle of this range. And remember, we’ve done the calculation for the part of each string that’s moving the most. Near its ends, each string will be moving much more slowly than this.

Complication 1 – how long is the round trip really?

So far, we’ve assumed that each part of the string does a simple back-and-forth movement along a straight line, but if you carefully watch a vibrating guitar string you’ll see that the string often moves in an irregular but roughly elliptical orbit. The wire-wound lower strings show this most clearly; you can see a hint of it in the image at the top. This makes the round-trip distance a bit longer than the 10 mm that we used in the calculation earlier. Does this affect the string’s average speed much?

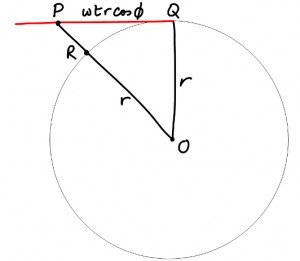
We’ll take the extreme case where each part of the string moves at constant speed in a circle of diameter 5 mm rather than along a straight line 5 mm long. The circumference of this circle will be $5 \pi$ mm, or about 15 mm. So the speeds of the strings (in this rather unlikely extreme case) will be about 50% greater than the ones listed above. They are still hardly impressive.

Complication 2 – the peak speed

So far, we’ve calculated the mean (average) speed of the string over its round trip. However, unless it’s moving in a perfect circle, its speed changes constantly, and its peak speed will be higher than its mean speed. How much higher?

Imagine that part of the string is vibrating back and forth along a straight line in the simplest possible way. At one end of the movement the string is stationary as it changes direction. It then speeds up, reaching its peak speed at the centre of its range of movement. Then it slows down until it reaches a halt again at the other end of the movement and changes direction again. How do we calculate the peak speed if we know the time taken for the round trip?

You’ll need to know a bit of maths for the next bit. The simplest vibration of the string is where each part undergoes simple harmonic motion, that is, where its position varies sinusoidally with time. This means in turn that the velocity of the string also varies sinusoidally in time. So we need to ask: how does the mean value of a sinusoid compare to its peak value?

Consider the function $y = \sin \theta$ , for half a cycle, that is, for theta from $0$ to $\pi$ radians. We construct a rectangle between these limits, such the rectangle’s area equals the area under the sine curve. The mean value of the sine function is the height of the rectangle, which its area divided by its width (which is $\pi$ ). So we need to work out the area $a$ under the sine curve between $0$ and $\pi$ , which we can do by integration:

$a = \int\limits_0^\pi \sin \theta \,d\theta$

$= \left[-\cos \theta\right]_0^\pi$

$= \left[-(-1)-(-1)\right]$

$= 2$

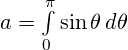
We constructed the rectangle to have area $a$ . Its width is $\pi$ , so its height, and therefore the mean value of the sine function, is $a/\pi = 2/\pi$ . The height to the peak of the sine curve is 1, so the peak value of the sine function is $\pi/2$ times its mean value. This means that the peak speed of our guitar string is $\pi/2$ times, or 50% more than, its average speed. Again, nothing to write home about.

(Rather pleasingly, this ratio of $\pi/2$ is the same ratio that we got earlier in Complication 1. It means that the peak speed of a particle doing simple harmonic motion with a given amplitude $r$ and period $t$ is exactly the same as the (constant) speed of a particle moving in a circular orbit of radius $r$ with period $t$ . This isn’t a coincidence. It arises because the circular motion can be considered as two linear simple harmonic motions at right angles to each other.)

Credits

“Vibrating guitar string” by jar [0] is licensed under CC BY 2.0

Mathematical typesetting by QuickLaTeX

A creative atmosphere

It’s sometimes said that if you sit an immortal monkey in front of an equally durable typewriter and leave it to tap randomly away at the keys, then eventually it will produce the entire text of Richard III (or any other Shakespeare play of your choice), completely by chance. All you have to do is wait long enough.

I was thinking about this one day, and also thinking about air molecules. In the room I’m sitting in at the moment, there are at least 1,000,000,000,000,000,000,000,000,000 air molecules, all frantically dashing around bumping into each other. How often, I wondered, do little clusters of these molecules fleetingly arrange themselves, by chance, in arrangements that we would regard as being somehow regular or remarkable?

It’s not possible for me to directly observe air molecules, so instead I used my computer to make a 2-dimensional simulation of some atoms of gas doing what atoms of gas do. I set it going, and waited…and waited…and waited…

I promise you that my simulation didn’t involve any secret forces drawing atoms towards certain positions. The movements and collisions of the atoms all occurred in accordance with the laws of mechanics. But I did cheat a little. Can you figure out how?

Molecules and atoms

In case you’re fidgeting on your chair wondering why I started talking about air molecules but finished talking about gas atoms, let me explain.

Nearly all of the air is nitrogen and oxygen. Nitrogen and oxygen atoms are essentially spherical. But in the air, nitrogen atoms are bonded together in pairs to form nitrogen molecules that are, roughly speaking, a stubby rod shape. The same goes for oxygen. Now a collision between two moving rods is much more complicated than a collision between two spheres, because the rods can spin end-over-end in a way that spheres can’t. In fact, I’m not sure that I know how to do the calculations. As the point of the video could be made just as well using atoms rather than molecules, I did the simulation using atoms. If you like, you can think of them as atoms of helium or argon, which do go around on their own.

If I suddenly became weightless, what would happen to me?

Suppose that you were standing perfectly still, and gravity suddenly stopped operating on your body. What would happen? Nothing much, you might think, apart from a queasy feeling of weightlessness. After all, an object won’t start to move unless a force acts on it, and no force is acting on your body.

nogravity1 — Diagram of the Earth, looking straight down on the north pole.

However, when you stand “still”, in fact you’re travelling in a very large circle at rather high speed, as the Earth turns on its axis and carries you with it. Newton’s 1st Law tells us that things travel in straight lines unless a sideways force acts upon them. The force that keeps tugging you to make you travel in a circle rather than in a straight line is gravity. This means that the moment gravity stops acting on you, you’ll start moving along a straight line (the red line in the diagram) while the ground continues to move in a circular path underneath you (the blue line).

The consequence is that you’ll lose contact with the Earth and float upwards, serenely or otherwise. At least, that’s what it will look like to earthbound observers. But what’s really happening is that the ground is accelerating downwards away from you as it moves on its curved path. Try to remember that as you watch your footprints receding beneath you.

I wondered how fast this would all happen. The answer is: remarkably quickly. I give the geometry later, for those who are interested, but here are some example results for a person standing in Edinburgh, on a latitude of 56° N. Just for now, we’ll pretend that there isn’t any air.

After 1 second your feet will be 5 millimetres off the ground.
After 10 seconds you’ll be 53 centimetres off the ground.
After a minute, you’ll be 19 metres up
After an hour you’ll be at an altitude of over 68 km (though, being half frozen to death by now, you may be losing interest).

The lower your latitude, the quicker your ascent. At the equator, you’ll rise about three times as fast as in Edinburgh, and at the poles, you won’t lose contact with the ground at all.

Why on earth should I be interested in a situation that is contrived and physically impossible? It’s because it brings home the fact that each of us is constantly moving along a curved path as the Earth rotates. On the equator, it takes only 10 seconds for our trajectory to deviate from a straight line by 1.7 metres (during which time we’ve travelled 465 metres).

Why did I pretend that there isn’t any air? Because the presence of air muddies the waters by adding another force: the upward force of our buoyancy in the air. At low altitudes this force isn’t negligible: it slightly more than doubles the first three figures above. As you rise further and the air gets thinner, it matters less and less. I left it out because I wanted to make the effect of the Earth’s rotation clear.

The question that I’ve just answered is a trimmed-down version of a question that my friend Malcolm and I occupied ourselves with once when we were on a rather long and boring tramp along a glen at the end of a camping trip in the Cairngorms in Scotland. The question we asked then was: what would we observe if gravity suddenly stopped operating altogether? I may return to that subject in a future post.

The geometry

Let the centre of the Earth be at $O$ , the origin, and let the Earth’s radius be $r$ and its angular velocity about its own axis be $\omega$ . You are standing at latitude $\phi$ and are therefore a distance $r \cos \phi$ from the Earth’s axis. Your linear velocity as you stand still on the rotating Earth will be $\omega r \cos \phi$ , tangential to the Earth’s surface.

Suppose that gravity stops acting on you at time zero, when you are at point $Q$ . With no gravity acting on you, will now travel in a straight line tangential to the Earth’s surface. The diagram shows the situation from a suitable vantage point, looking sideways on to your direction of travel. We are not looking down on the north pole.

After a time $t$ , you will have travelled a distance $\omega t r \cos \phi$ , to point $P$ .

Your altitude is the distance $PR$ , where $R$ is the point on the Earth’s surface for which $P$ is directly overhead. $R$ lies on $OP$ , the line from $P$ to the centre of the Earth. The length of $OP$ is given directly by Pythagoras’ Theorem in triangle $OPQ$ : it’s $\sqrt{(\omega t r \cos \phi)^2 + r^2}$ . As $OP=PR+r$ , the altitude of point $P$ is $OP - r$ . So

$PR = ((\omega t r \cos \phi)^2 + r^2)^{1/2} - r$

All we need now is $\omega = 2\pi/86400 \text{ s}^{-1}$ , because the Earth does one full rotation in 86400 seconds, and $r=6.4\times10^6 \text{ m}$ , because that’s how big the Earth is. We can now choose $\phi$ and calculate $PR$ for any value of $t$ .

We can check this answer in two ways. Firstly, we can use the very useful intersecting chords theorem to calculate the distance marked $h$ in the diagram on the right. For small values of $t$ , where $h \ll r$ , then $h$ should be approximately equal to the your altitude $PR$ . For values of t of 1, 10, or 60 seconds, $h$ and $PR$ agree to 4 significant figures. As we expect, as $t$ increases, the agreement gets less good: $h$ and $PR$ differ by about 2% after 1 hour.

The second check is to differentiate the expression for $PR$ twice with respect to time. The first differentation gives us an expression for the rate of change of $PR$ with respect to time, that is, your rate of gain of altitude:

$\frac{dPR}{dt} = \frac{\omega^2rt}{ (1+\omega^2t^2)^{1/2}}$

(Note: to keep things clear, I’ve omitted $\cos \phi$ , which merely accompanies $r$ everywhere and doesn’t change the conclusions.) Where $t=0$ , and your motion is purely tangential, this expression for your speed away from the ground should be zero, and where $t$ is very large $(\omega t \gg 1)$ , and your motion is purely radial, your speed away from the ground should be $\omega r$ . Both are true.

The second differentiation gives us an expression for your upward radial acceleration:

$\frac{d^2PR}{dt^2} = \frac{\omega^2r}{(1+\omega^2t^2)^{3/2}}$

As it’s not you accelerating, but the ground that is accelerating away from you as it continues on its circular path, this expression for your upwards acceleration should, where $t=0$ and your path is tangential to the surface, become the same as that for the centripetal acceleration of the ground, $\omega^2 r$ , which it does. In addition, when $\omega t r \gg r$ , and your path is almost radial, the expression for your acceleration should approach zero, which it does.